| |
|
In Infinity number 19 we gave you a Sudoku puzzle (which used the nine symbols L,A,T,I,N,S,Q,R,E instead of the nine digits 1,2,…,9). A puzzle which is becoming almost as popular as Sudoku is known as KAKURO (or Cross Sums, or Kakro, as it’s called in different countries).
For this sort of puzzle we must use the nine digits 1, 2, …, 9, rather than nine other letters or symbols, because they retain their meaning, and we need to be able to add up different combinations of distinct digits! Here is a small Kakuro puzzle to illustrate what is needed:
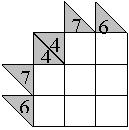
The aim of the puzzle is to fill the empty cells with digits from 1, 2, …, 9 so that the sum of the numbers in each row and each column is equal to the given number clue, and no digit is repeated in any answer. A number clue in the bottom triangle of a header cell tells you the required sum of the cells below it and a number clue in the top triangle of a header cell tells you the required sum of the cells to the right of it.
Since each sum must involve distinct digits, two cells summing to 3 must be 1, 2 or else 2, 1; and two cells summing to 4 must be 1, 3 or 3, 1. We can’t use 4 = 2 + 2 because the digits used must all be distinct! Similarly, three cells summing to 6 must be filled with 1, 2, 3 in some order, and three cells summing to 7 must be filled with the digits 1, 2, 4 in some order.
In the above puzzle, the digit 3 in the row to the right of the clue 4 must be under the clue 6 (since it can’t lie in the column labelled 7). Thus the two cells in the first row are 1 and 3 in that order.
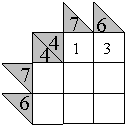
Then the middle cell in the column labelled 7 and the row labelled 7 must be 4, since that row must contain 1, 2, 4 in some order and 4 cannot appear under the label 6 and cannot appear under the label 4. Also, the cell in the row labelled 7 and the column labelled 4 must be 1 since we can’t have 4 = 2 + 2. The rest follows easily.

For larger numbers, there are often unique ways to fill a small number of cells (apart from the order in which the digits appear; e.g. 17 = 8 + 9 = 9 + 8). You could make a list of “unique” sums to refer to when completing a Kakuro puzzle:
| Two cells: | Three cells: | Four cells: |
| 3 = 1 + 2 | 6 = 1 + 2 + 3 | 10 = 1 + 2 + 3 + 4 |
| 4 = 1 + 3 | 7 = 1 + 2 + 4 | 11 = 1 + 2 + 3 + 5 |
| 16 = 7 + 9 | 23 = 6 + 8 + 9 | 29 = 5 + 7 + 8 + 9 |
| 17 = 8 + 9 | 24 = 7 + 8 + 9 | 30 = 6 + 7 + 8 + 9 |
Now try your hand at this Kakuro puzzle!

Click here for the Answer.
|
|
|




