|
||
|
The strands of DNA that we see are often knotted. However the structure of the knots is always changing. When a cell is copying a DNA strand it will often uncoil and recoil a strand, then cut it and knot it. In addition, enzymes called topoisomerases will often attach themselves to a closed loop of DNA, break the molecular bond, and then twist the two ends of the DNA in opposite directions before rejoining the ends and floating away.
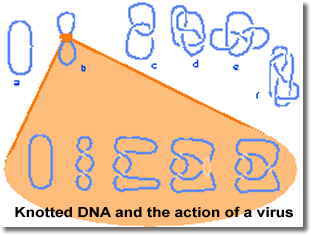
Certain viruses entering the nucleus of a cell encase a section of the DNA in a ball of enzyme, cutting and twisting the DNA and inserting viral DNA into the host organism. The electron micrograph given on the left illustrates a loop of DNA which is being attacked by a virus. You can see where a section of the DNA is encased by a virus. Initially the DNA formed a single loop, but the virus mutates the DNA to look like one of the structures shown in Figure 1.Unfortunately biologists cannot enlarge the enzyme ball enough to identify the exact changes occurring within it. But by working with mathematicians, knowledgeable in knot theory, they have been able to work out what is happening. By modeling the DNA as a string with knots in it (see figure) we can show that knot a) can be transformed to knot c) by twisting the strands, breaking them and then rejoining the strands together. In addition repeating this process results in knots d), e) and f), indicating that this is the mechanism by which viruses change the DNA. (See Lifting the curtain: using topology to probe the hidden action of enzymes, by De Witt Sumners, Notices of the American Mathematical Society, 42, May 1995.) DNA is just one application of Knot theory, which is presently an area of intense mathematical activity worldwide. The Knot theory group at the Department of Mathematics, the University of Queensland (including Mark Gould, Tony Bracken, Jon Links and David McAnally) use algebraic group theory to classify knots and identify such things as mutant knots!
|
||
 |